Physics CIRCULAR MOTION of a Car on a road

Please Wait... While Loading Full Video

CLASS 11 CHAPTER 5 - LAWS OF MOTION

CIRCULAR MOTION of a Car on a road

Topics Covered

• Circular Motion
• Motion of a Car on a Level Road
• Motion of a Car on a Banked Road

Circular Motion

`\color{red} ✍️` We know that the acceleration of a body moving in a circle of radius `color{blue}{R}` with uniform speed `color{blue}{v}` is `color{red}{v^2//R)` directed towards the centre.

According to the second law, the force `f` providing this acceleration is :

`color{red}{f=(mv^2)/R}`

where `m` is the mass of the body.

• This force directed forwards the centre is called the centripetal force.

• For a stone rotated in a circle by a string, the centripetal force is provided by the tension in the string. The centripetal force for motion of a planet around the sun is the gravitational force on the planet due to the sun.

• For a car taking a circular turn on a horizontal road, the centripetal force is the force of friction. The circular motion of a car on a flat and banked road give interesting application of the laws of motion.

Motion of a car on a level road

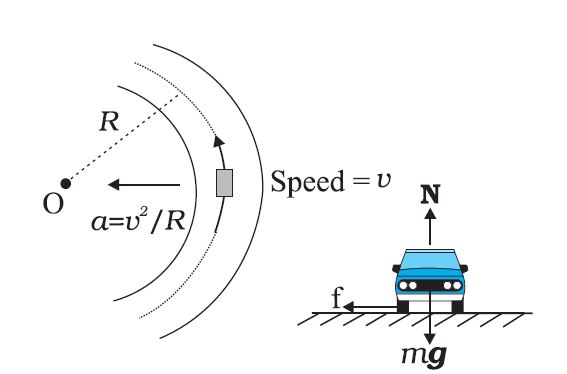
Three forces act on the car. (Fig. 5.14(a)

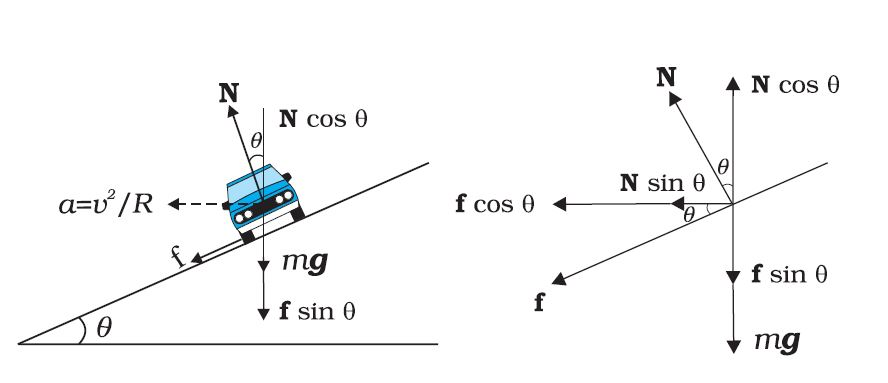
`(i)` The weight of the car, `color{red}{mg}`

`(ii)` Normal reaction, `color{red}{N}`

`(iii)` Frictional force, `color{red}{f}`

As there is no acceleration in the vertical direction
`N–mg = 0`

`color{blue}{N = mg}`

The centripetal force required for circular motion is along the surface of the road, and is provided by the component of the contact force between road and the car tyres along the surface. This by definition is the frictional force.

Note that it is the static friction that provides the centripetal acceleration. Static friction opposes the impending motion of the car moving away from the circle.

`f <= mu_s N = (mv^2)/R`

`v^2<=(mu_sRN)/m=mu_s Rg` [`N=mg`]

which is independent of the mass of the car.

`color{green}{=>"Maximum speed of circular motion"} color{red}{\ \v_(m a x) = sqrt(mu_s Rg)}`

Q 3250467314

A cyclist speeding at 18 km/h on a level road takes a sharp circular turn of radius 3 m without reducing the speed. The co-efficient of static friction between the tyres and the road is 0.1. Will the cyclist slip while taking the turn ?

Solution:

On an unbanked road, frictional force alone can provide the centripetal force needed to keep the cyclist moving on a circular turn without slipping. If the speed is too large, or if the turn is too sharp (i.e. of too small a radius) or both, the frictional force is not sufficient to provide the necessary centripetal force, and the cyclist slips. The condition for the cyclist not to slip is given by Eq. (5.18) :

`color{green}{v^2 <= μ_s R g}`

Now, `R = 3 m, g = 9.8 m s^-2, μ_s = 0.1`.

That is,

`μ_s R g = 2.94 m^2 s^-2`. v = 18 km/h `= 5 m s^-1`; i.e.,

`v^2 = 25 m^2 s^-2`.

The condition is not obeyed.
The cyclist will slip while taking the circular

Q 3260467315

A circular racetrack of radius 300 m is banked at an angle of 15°. If the coefficient of friction between the wheels of a race-car and the road is 0.2, what is the (a) optimum speed of the racecar to avoid wear and tear on its tyres, and (b) maximum permissible speed to avoid slipping ?

Solution:

On a banked road, the horizontal component of the normal force and the frictional force contribute to provide centripetal force to keep the car moving on a circular turn without slipping. At the optimum speed, the normal reaction’s component is enough to provide the needed centripetal force, and the frictional force is not needed. The optimum speed vo is given by Eq. (5.22):

`v_o = (R g tan theta)^(1/2)`

Here R = 300 m, `theta = 15°, g = 9.8 m s^-2`; we
have
`v_o = 28.1 m s^-1`.

The maximum permissible speed vmax is given by Eq. (5.21):

`v_(max) = (Rg (mu_s + tan theta )/( 1 - mu_s tan theta ))^(1/2) = 38.1 ms^-1`

Motion of a car on a banked road

We can reduce the contribution of friction to the circular motion of the car if the road is banked (Fig. 5.14(b)). Since there is no acceleration along the vertical direction, the net force along this direction must be zero. Hence,

`N cos θ = mg + f sin θ`.....(i)

The centripetal force is provided by the horizontal components of N and f.

`N sin θ + f cos θ = (mv^2)/R`......(ii)

But `f_s <= mu_s N`, thus to obtain `v_(max)` we put `f = mu_s N`

From equation (i) and (ii)

`N cos θ = mg + mu_s N sin θ`.....(iii)

`N sin θ + mu_sN cos θ = (mv^2)/R`.....(iv)

We obtain, `color(blue)(N=(mg)/(cosθ - mu_s sinθ))`

Substituting value of `N` in Eq. (iv), we get

`(mg(sin theta - mu_s cos theta))/(cos theta - mu_s sin theta) = (mv_(max)^2)/R`

`color(red)(v_(max) = (Rg (mu_s + tan theta)/(1- mu_s tan theta))^(1//2))`

For `color{blue}{mu_s =0}`, `color(red)(v_o = sqrt(Rg tan theta))`

At this speed, frictional force is not needed at all to provide the necessary centripetal force. Driving at this speed on a banked road will cause little wear and tear of the tyres.

The same equation also tells you that for `v < v_o`, frictional force will be up the slope and that a car can be parked only if `tan θ ≤ mu_s`.

Solving Problems in Mechanics

To handle a typical problem in mechanics systematically, one should use the following steps :

`(i)` Draw a diagram showing schematically the various parts of the assembly of bodies, the links, supports, etc.

`(ii)` Choose a convenient part of the assembly as one system.

`(iii)` Draw a separate diagram which shows this system and all the forces on the system by the remaining part of the assembly. Include also the forces on the system by other agencies. Do not include the forces on the environment by the system. A diagram of this type is known as ‘a free-body diagram’.

`(iv)` In a free-body diagram, include information about forces (their magnitudes and directions) that are either given or you are sure of (e.g., the direction of tension in a string along its length). The rest should be treated as unknowns to be determined using laws of motion.

`(v)` If necessary, follow the same procedure for another choice of the system. In doing so, employ Newton’s third law. That is, if in the free-body diagram of A, the force on A due to B is shown as `vecF`, then in the free-body diagram of B, the force on B due to A should be shown as `–vecF`.

Q 3270467316

See (Fig. 5.15) A wooden block of mass 2 kg rests on a soft horizontal floor. When an iron cylinder of mass 25 kg is placed on top of the block, the floor yields steadily and the block and the cylinder together go down with an acceleration of `0.1 m s^-2`. What is the action of the block on the floor (a) before and (b) after the floor yields ? Take `g = 10 m s^-2`. Identify the action-reaction pairs in the problem.

Solution:

(a) The block is at rest on the floor. Its free-body diagram shows two forces on the block, the force of gravitational attraction by the earth equal to `2 × 10 = 20 N`; and the normal force R of the floor on the block. By the First Law, the net force on the block must be zero i.e., R = 20 N. Using third law the action of the block (i.e. the force exerted on the floor by the block) is equal to 20 N and directed vertically downwards.

b) The system (block + cylinder) accelerates downwards with `0.1 m s^-2`. The free-body diagram of the system shows two forces on the system : the force of gravity due to the earth (270 N); and the normal force R¢ by the floor. Note, the free-body diagram of the system does not show the internal forces between the block and the cylinder. Applying the second law to the system,

`270 – R' = 27 × 0.1N`

ie. `R' = 267.3 N`

By the third law, the action of the system on the floor is equal to 267.3 N vertically downward.

Action-reaction pairs :

For (a): (i) the force of gravity (20 N) on the block by the earth (say, action); the force of gravity on the earth by the block (reaction) equal to 20 N directed upwards (not shown in the figure). (ii) the force on the floor by the block (action); the force on the block by the floor (reaction).

For (b): (i) the force of gravity (270 N) on the system by the earth (say, action); the force of gravity on the earth by the system (reaction), equal to 270 N, directed upwards (not shown in the figure).

(ii) the force on the floor by the system (action); the force on the system by the floor (reaction). In addition, for (b), the force on the block by the cylinder and the force on the cylinder by the block also constitute an action-reaction pair.

The important thing to remember is that an action-reaction pair consists of mutual forces which are always equal and opposite between two bodies. Two forces on the same body which happen to be equal and opposite can never constitute an action-reaction pair. The force of gravity on the mass in (a) or (b) and the normal force on the mass by the floor are not actionreaction pairs. These forces happen to be equal and opposite for (a) since the mass is at rest. They are not so for case (b), as seen already. The weight of the system is 270 N, while the normal force R¢ is 267.3 N.